Journal of Financial Planning: June 2013
Executive Summary
- The safety of a 4 percent initial withdrawal strategy depends on asset return assumptions. Using historical averages to guide simulations for failure rates for retirees spending an inflation-adjusted 4 percent of retirement date assets over 30 years results in an estimated failure rate of about 6 percent. This modest projected failure rate rises sharply if real returns decline.
- As of January 2013, intermediate-term real interest rates were about 4 percent less than the historical average used in previous simulations. Calibrating bond returns to the January 2013 yields offered on five-year TIPS to match the duration of bond investments used in previous simulations, while maintaining the historical equity premium, causes the projected failure rate for retirement account withdrawals to jump to 57 percent. Results from this analysis suggest that the 4 percent rule cannot be treated as a safe initial withdrawal rate.
- Some financial planners may wish to assume that today’s low interest rates are an aberration and that higher real interest rates will return in the medium-term horizon. Although there is little evidence to support this assumption, we estimate how a reversion to historical real yields will affect failure rates.
- Because of sequence of returns risk, portfolio withdrawals can cause the events in early retirement to have a disproportionate effect on the sustainability of an income strategy. We simulate failure rates if today’s bond rates return to their historical average after either five or 10 years and find that failure rates are much higher (18 percent and 32 percent, respectively, for a 50 percent stock allocation) than many retirees may be willing to accept.
- The success of the 4 percent rule in the United States may be a historical anomaly, and clients may wish to consider their retirement income strategies more broadly than relying solely on systematic withdrawals from a volatile portfolio.
Michael Finke, Ph.D., CFP®, is a professor and Ph.D. coordinator in the Department of Personal Financial Planning at Texas Tech University. (Michael.Finke@ttu.edu)
Wade D. Pfau, Ph.D., CFA, is a professor of retirement income at the American College. (WadePfau@gmail.com)
David M. Blanchett, CFP®, CFA, is head of retirement research at Morningstar Investment Management. (David.Blanchett@morningstar.com)
At the start of 2013, real bond yields were much lower than historical averages. Investors in inflation-protected Treasury bonds (TIPS) were willing to accept a negative real return on bond investments for maturities below 20 years, which is a period of negative real yields longer than any that has occurred in the United States. Treasury rates of return have been, and as of this writing are, below current and projected near term inflation rates; even the nominal rate of return on 10-year Treasuries is below 2 percent.
For data used in pioneering studies of safe withdrawal rates using historical rolling time periods (Bengen 1994; Cooley, Hubbard, and Walz 1998), the average real return on bonds was 2.6 percent. Previous analyses did not include a long period of low real bond yields. Bengen focused on the worst case scenario in history, in which a retiree in 1966 could sustainably support an inflation-adjusted withdrawal amount of just more than 4 percent of retirement date assets over the subsequent 30 years. Interestingly, from 1966, the average real bond returns over the subsequent five, 10, and 30 years were 0.7 percent, 0.15 percent, and 3.1 percent, respectively.
Cooley, Hubbard, and Walz (1998) later introduced the concept of failure rates within the historical data, which shows how often in history a strategy would have failed. Because these calculations were not based on current market conditions, their usefulness and relevance is not clear for clients seeking forward-looking success rates for their retirement strategies.
Sustainable retirement withdrawal simulations that use the Monte Carlo analysis technique also have assumed fluctuations around the historical real rate of return on bond and stock investments within a portfolio without further questioning whether this is a relevant assumption. Probably the best demonstration of this particular Monte Carlo simulation approach was presented by Spitzer, Strieter, and Singh (2007). They provided illustrations for how withdrawal rates, asset allocations, failure probabilities, and bequest motives all interact for a 30-year retirement.
Financial planners faced with low current bond yields have two principal choices if they want to follow a traditional safe withdrawal strategy in retirement. The first is to assume that the current market price is the best estimate of future bond yields. This assumption allows for safe withdrawal rate simulations in a low-yield environment. There is convincing empirical evidence that today’s return is a consistent predictor of 10-year returns, and that the first 10 years of returns in retirement have a disproportionate impact on retirement failure rates (Milevsky and Abaimova 2006; Pfau 2011b).
The second is to assume that low bond yields are an aberration and that higher real yields eventually will return. The implicit assumption is that bond returns are mean reverting and likely will increase in the future. There is very little evidence to support this scenario in the literature. In this paper we do, however, estimate what will happen to retirement income failure rates if real yields go back up. Our results suggest that even relying on rosier future yield assumptions is not a panacea that will dramatically improve the safety of an inflation-adjusted withdrawal amount calibrated to 4 percent of retirement date assets.
The primary objective in this paper is to explore what happens if it is assumed that today’s interest rates reflect expectations of future bond returns within a retirement portfolio. That is, if we assume that the future real bond return for today’s retirement portfolios is near zero and financial planners follow the traditional withdrawal rate methodology that has given us the 4 percent rule, what withdrawal rate will be safe for today’s retirees? In other words, what percentage of retirement date assets can be spent, with that amount adjusted for inflation in subsequent years, to provide sustainability over a 30-year retirement with a sufficiently high probability based on today’s yields? With equity market returns corrected for these lower risk-free rates, we will provide scenarios with flat real bond yields and with current negative real yields.
Can We Rely on Rising Bond Rates?
Using historical data assumes that the mean and standard deviation of future bond returns will resemble the past. However, the fundamentals of bond supply and demand today suggest that a reversion to much higher real bond rates is unlikely. To understand why, it is important to review how bond prices are formed.
A basic bond pricing model is the so-called Fisher equation that decomposes the bond interest rate into two basic features: the real rate of interest and inflation expectations. Current low interest rates can be seen as extremely low inflation expectations, a very low real yield, or both. Fortunately, TIPS eliminate any consideration of inflation expectations because their values rise and fall to counteract changes in periodic inflation rates. It is possible to look at current and future TIPS rates to place a market value on the current real rate of return on bonds.
The real rate of return with financial markets represents the marginal investor’s willingness to trade consumption over time. In other words, when they give up $1 in spending today by buying a bond, how much additional purchasing power will they receive in the future? Current rates on TIPS indicate that the market is essentially trading purchasing power across time without any real premium for deferred consumption for maturities up to 20 years. Put another way, investors are willing to give up a dollar today to receive a dollar’s worth of inflation-protected spending in 20 years.1 They are willing to give up a dollar in spending today to receive 94 cents worth of spending in 10 years, based on the future value of one dollar invested at current 10-year TIPS rates issued January 31, 2013.
Low yields on bonds appear to be the result of excess demand fueled by the demographics of an aging population and the growth of economies with higher average savings rates (Arnott and Chaves 2012). As populations continue to age, it is likely that demand for fixed income securities and equities will continue to be strong (Bakshi and Chen 1994). Governments also have increased the supply of bonds, adding to national debt among many developed countries.
Federal Reserve Chairman Ben Bernanke recently noted that “keeping inflation close to 2 percent will likely require real short-term rates, currently negative, to remain low for some time” (Bernanke 2013). A decomposition of 10-year Treasury bond rates indicates that markets anticipate real short-term rates over the next decade to be near or below zero. Feldstein (2013) argued that the Federal Reserve has contributed to low nominal rates through open market bond purchases; however, any possible rise in nominal rates, as a result, likely will be accompanied by inflation rather than real yields, as well as a decline in real equity returns (Feldstein).
There also is some dispute about whether monetary policy has much impact on nominal rates because nominal (and real) 10-year government bond rates have been nearly identical in the United Kingdom, Japan, Germany, Canada, and the United States since 2000 (Bernanke 2013).
An investor may reject the hypothesis that demographic and macroeconomic factors will keep real rates low. Beyond this, there is little empirical evidence that bond yields do tend to revert back to the mean. An early model theory of bond mean reversion introduced by mathematician Vasicek (1977) suggests that interest rates tend to bounce around a mean historical long-run trend. There are three components of this model: the long-run trend itself, drift, and dispersion. Retirees today should be most interested in the drift concept. Think of the historical interest rate average as a magnet attracting extreme interest rates back to the central tendency. Each year, interest rates vary according to some random effect, the size of which is part of the process and can be estimated with a historical standard deviation, but generally move back in the direction of the mean. The drift component means that tomorrow’s interest rates are likely to look exactly like today’s interest rates within a band of randomness. But the general direction of that drift is likely going to be toward the mean. The best guess of tomorrow’s interest rates is whatever they are today—plus or minus a certain amount of random movement—but in the general direction of the historical average.
In a recent review of the evidence on interest rate mean reversion using 200 years of data across four countries, including the United States (van den End 2011), there appears to be little evidence that bonds consistently revert back to their historical average in any predictable manner. In other words, interest rates can be higher or lower than the average for long periods and it is impossible to predict when they will return to the mean. Evidence of the persistence of nominal bond rates can be found by simply comparing the relation between current yields and subsequent returns in the United States. Figure 1 shows the relationship between bond yields and the future average annualized return on bonds using the Ibbotson Intermediate-Term Government Bond (ITGB) index as a proxy for bonds.
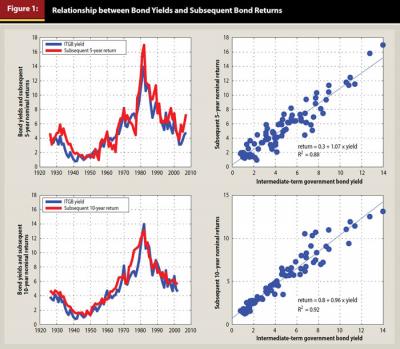
The historical relation between bond yields and the future average annualized return on bonds has been quite strong, with a coefficient of determination (R²) of 88 percent for subsequent five-year returns, and 92 percent for subsequent 10-year returns. This means that the yield on bonds today can describe 92 percent of variation in the average annual 10-year compounded bond return. If we assume a current bond yield of 0.7 percent (which was the nominal yield on a five-year constant maturity nominal Treasury bond at the start of January 2013), the average annualized bond return is expected to be 1.05 percent over the next five years, and 1.4 percent over the next 10 years using the linear regression model in Figure 1.
This is considerably less than the average nominal return of 5.5 percent for the ITGB index since 1926. It is also likely to be considerably less than inflation, as the best estimates for inflation can be found by comparing constant maturity Treasury yields and TIPS yields. At the start of January, these breakeven inflation rates were 2.1 percent over the next five years (five-year Treasury of 0.7 percent and five-year TIPS of −1.4 percent) and 2.5 percent over the next 10 years (10-year Treasury of 1.9 percent and 10-year TIPS of −0.6 percent).
While increasing bond yields would result in higher returns for new bond investors, it would negatively affect those currently holding bonds. One method to approximate the impact of a change in interest rates on the percentage change in the price of a bond is to multiply the bond’s duration by the percentage change in interest rates times negative one. For example, if interest rates increase by 2 percent, a bond with a duration statistic of five years (the approximate current duration of the Barclays Aggregate Bond Index) would decrease in value by 10 percent. The impact on bonds with longer durations (for example, 15 years) obviously would be even more extreme.
Return Assumption Sensitivity
To illustrate the sensitivity of failure rates to changes in yield assumptions, failure rates using current market yields are shown in Figure 2. Using historical data, Bengen (1994) showed that the 4 percent rule had always worked in overlapping 30-year historical periods using an asset allocation of 50 percent large-capitalization stocks and 50 percent intermediate-term (five-year) bonds.
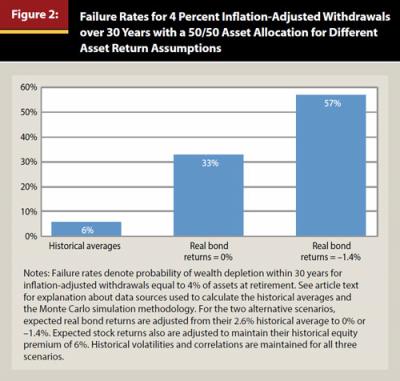
With Monte Carlo simulations based on the same historical data, the increasing number of simulated runs (compared to the 57 rolling 30-year historical periods now available since 1926) tend to result in a failure rate—the percentage of cases in which withdrawals are not sustainable for 30 years—of about 6 percent for the same retirement strategy (Pfau 2011c). These Monte Carlo simulations are based on average real bond returns of 2.6 percent and average real stock returns of 8.6 percent. If bond and stock returns are adjusted downward by 2.6 percent (this maintains the same historical average premium that stocks earned over bonds), the failure rate jumps to 33 percent. And when bond returns are calibrated to the January 2013 real yields offered on five-year TIPS, to be consistent with prior studies that use backward-looking five-year bond returns, while maintaining the historical equity premium, the failure rate jumps to 57 percent.
Although future bond returns are random, the historical relation between current and future bond yields suggests that the 4 percent rule cannot be treated as a safe initial withdrawal rate in today’s low interest rate environment.
Next, we discuss the implications of the second scenario, which is that of bond yields increasing in the future, allowing higher sustainable withdrawals today. To do this, we simulated the impact of an increase in real rates to their historical average. But because of sequence of returns risk, research shows that a future climb in real interest rates is not as promising as one might suspect. If real bond returns center around −1.4 percent for 10 years and then revert to the historical 2.6 percent average, the failure rate drops from 57 percent to 32 percent. Interestingly, if the interest rate reversion happens in five years, the failure rate is still 18 percent. In both cases, these failure rates are significantly higher than when using historical averages for the entire retirement period. These failure rate estimates are really best case scenarios for a future interest rate rise, as they do not incorporate any capital losses that many retirees would experience on their medium- and long-term bond holdings if real interest rates were to suddenly rise by 4 percent.
Assuming that interest rates will rise in the medium term is not a valid justification for using historical averages when simulating retirement outcomes, because asset returns during the first few years of retirement have an overwhelming impact on eventually running out of money. Below-average returns cause retirees to consume a larger percentage of their portfolios earlier in retirement, leaving fewer assets available with which to capture any subsequent market rebounds. This is why Bengen (1994) found that the safe withdrawal rate is less than the average portfolio return. Pfau (2011b) estimated that the cumulative portfolio returns and inflation in the first 10 years of retirement explain about 80 percent of final retirement outcomes.
Safe Withdrawal Rate Studies
It is possible to classify existing withdrawal rate studies into several categories. First are studies that use overlapping periods from historical data. The most common historical data are Ibbotson Associates’ Stocks, Bonds, Bills, and Inflation (SBBI) data for total returns in U.S. financial markets since 1926. Studies of this nature tend to support a relatively high stock allocation in retirement and tend to provide the strongest support for the safety of the 4 percent rule. For instance, the seminal Bengen (1994) paper concludes that retirees in all historical circumstances could safely withdraw 4 percent of their assets at retirement and adjust this amount for inflation in subsequent years for a 30-year retirement. Using the S&P 500 and ITGB index, he determined that retirees are best served with a stock allocation between 50 percent and 75 percent, concluding “stock allocations below 50 percent and above 75 percent are counterproductive.” (Bengen 1994, 179)
A second approach to studying withdrawal rates is to use Monte Carlo simulations that are parameterized to the same data used in historical simulations. This can be done either by randomly drawing past returns from the historical data to construct 30-year sequences of returns in a process known as bootstrapping, or by simulating returns from a distribution (usually a normal or lognormal distribution) that matches the historical parameters for asset returns, standard deviations, and correlations. This simulation approach has the advantage of allowing for a greater variety of scenarios than the rather limited variety historical data can provide. Monte Carlo simulation studies usually show slightly higher failure rates for stock allocations in the 50 percent to 75 percent range. At the same time, if past returns do not reflect the distributions for future returns (for example, if the mean return on assets is lower) then these Monte Carlo simulations will overestimate the sustainability of various withdrawal rates.
We followed a third approach, which is to base Monte Carlo simulations on current market conditions rather than using historical averages. This approach reduces the requirement for forecasting skill. As illustrated in Figure 1, there is strong evidence that current bond yields consistently explain future bond returns. Because current intermediate-term real bond yields are 4 percent less than the historical average used in previous Monte Carlo studies, a cautious retiree must plan for lower bond and stock returns in the future, even if the equity premium for stocks maintains its historical average.
Some previous studies consider asset returns that are based on current market indicators rather than on historical averages. Blanchett and Blanchett (2008) made the point that future returns for a 60/40 portfolio could be one or two percentage points less than historical averages. They showed how portfolio failure rates relate to changes in both the assumed return and standard deviation.
Kitces (2008) explained how sustainable withdrawal rates can be linked to stock market valuation levels, and Pfau (2011a) demonstrated how sustainable withdrawal rates can be explained fairly well with a regression model using stock market valuations, dividend yields, and bond yields at the retirement date. Pfau (2012) provided a framework for planners to develop their own estimates for safe withdrawal rates based on their own capital market expectations and asset class choices.
Methodology and Data
The maximum sustainable withdrawal rate (MSWR) is the highest withdrawal rate that would have provided a sustained real income over a given retirement duration. At the beginning of the first year of retirement, an initial withdrawal is made equal to the specified withdrawal rate multiplied by accumulated wealth. Remaining assets then grow or shrink according to the asset returns for the year. At the end of the year, the remaining portfolio wealth is rebalanced to the target asset allocation. In subsequent years, the withdrawal amount adjusts by the previous year’s inflation rate and the order of portfolio transactions is repeated.
Withdrawals are made at the start of each year. Withdrawal amounts are not affected by asset returns, so the withdrawal amount as a percentage of remaining assets will vary from year to year as the portfolio fluctuates over the retirement period. If the withdrawal pushes the account balance to zero, the withdrawal rate was too high and the portfolio failed. The MSWR is the highest rate that succeeds. Taxes are not specifically incorporated and any taxes would need to be paid from the annual withdrawals. To be consistent with Bengen’s (1994) original study, we did not incorporate fees, suggesting that our results are more optimistic than otherwise would be the case. Monte Carlo simulations were performed using a lognormal distribution for 1 + return.
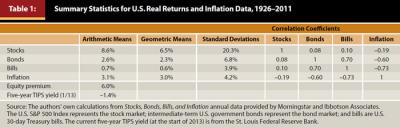
To consider how capital market expectations affect withdrawal rates and asset allocation, planners can identify the expected real arithmetic returns, standard deviations, and correlations between assets that they intend to include in their clients’ portfolios. Frequently, studies are based on historical data parameters. Table 1 provides this information for large-cap stocks, governments bonds, and Treasury bills for the years 1926 to 2011.
Our simulations investigate the impact of current market conditions, with expected returns adjusted for the most recent yield on five-year TIPS, a real asset with the same maturity as the ITGB index. The selection of bond yields with a five-year duration is consistent with the approach taken in the original Bengen (1994) study.2
Our assumption that risk-free bond rates are lower than the historical average also affects estimates of real equity yields, as stock returns are a combination of real risk-free returns and a risk premium according to the capital asset pricing model (CAPM). The historical real average equity return of 8.6 percent can be seen as a combination of the real return on risk-free assets (2.6 percent) and the equity premium (8.6 percent minus 2.6 percent, or 6.0 percent). Current risk-free real yields from TIPS are −1.4 percent. If the same 6 percent risk premium is projected for stocks, then the projected net real return from equity investments is 4.6 percent.3 If no real return on risk-free assets is projected, then the equity premium would be 6 percent.
In addition to maintaining the same return assumptions throughout retirement, we will consider cases in which current market conditions define the returns for the first part of retirement, and then returns revert back to their historical averages later in retirement. Such modifications make sense, because it is rather unlikely that −1.4 percent would continue as the average interest rate for five-year TIPS over an entire 30-year period. The purpose of doing this is to investigate the role of sequence of returns risk, and whether failure rates will increase dramatically even if historical averages return in five or 10 years. These estimates would really be the best case scenarios, because any increase in interest rates likely will result in capital losses for bond holdings that are not considered in the analysis.
Results
Figure 1 on page 48 summarizes the main results from this study. Table 2 provides more detailed information about failure rates for inflation-adjusted withdrawals set equal to 4 percent of retirement date assets (the 4 percent rule) over a 30-year period using several different asset allocations and capital market expectations. The stock allocations shown in Table 2 include 30 percent, 50 percent, and 70 percent. With the historical data, failure rates range between 6 percent and 8 percent.
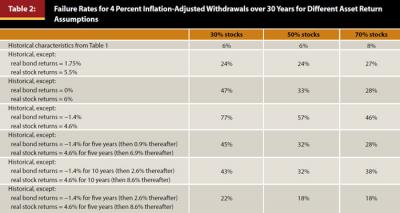
The most significant result of this research, however, is how dramatically failure rates can increase when return assumptions are reduced. For instance, return assumptions for a popular financial planning software program include average real bond returns of 1.75 percent and average real stock returns of 5.5 percent. With these assumptions, the failure rates for the selected asset allocations range from 24 percent to 27 percent. Moving closer to current conditions, if real bond returns are assumed to be 0 percent and the historical equity premium, failure rates are 47 percent for 30 percent stocks, 33 percent for 50 percent stocks, and 28 percent for 70 percent stocks. And considering the historical equity premium connected to current real bond yields, we estimate failure rates for the 4 percent rule of 77 percent for 30 percent stocks, 57 percent for 50 percent stocks, and 46 percent for 70 percent stocks.
Next in Table 2 are the results if TIPS average −1.4 percent for five years and then increase to 0.9 percent for the subsequent 25 years (with corresponding adjustments for stocks to maintain the same equity premium), to illustrate the market pricing for interest rates based on the current 30-year TIPS yield of 0.4 percent. Failure rates for the three stock allocations are 45 percent, 32 percent, and 28 percent.
The final two sets of assumptions in Table 2 begin with current market conditions and adjust upward to historical averages later in retirement. In the first example, historical averages guide the simulations after 10 years. In this case, failure rates range from 32 percent to 43 percent for the selected asset allocations.
In the final example, historical averages return after five years. In this case, failure rates range from 18 percent to 22 percent for the selected asset allocations. As mentioned earlier, these are really best case scenarios that do not account for additional potential market losses when interest rates rise. Yet, even if current market conditions only defined the first five years of retirement, failure rates are still dramatically higher than when the historical averages define the entire retirement.
Next, Figure 3 provides a more detailed investigation of the failure rates over a 30-year retirement for a variety of asset allocations and withdrawal rates using market condition assumptions of a −1.4 percent average real bond return and the historical equity premium.
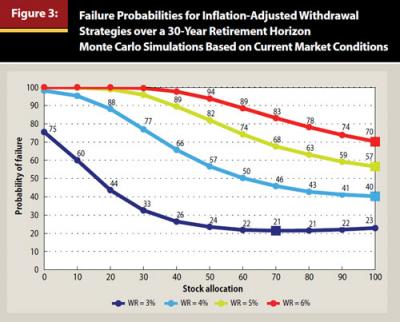
Squares are shown at the asset allocations that minimize the failure rate for each withdrawal rate. With these assumptions, even a 3 percent withdrawal rate has a more than 20 percent failure rate for all asset allocations. These results measure the probability of failure rather than the magnitude of failure, and with a 4 percent withdrawal rate, the probability of failure is minimized with 100 percent stocks. That failure rate is 40 percent. For a conservative client with less than 60 percent stocks, failure rates are more than 50 percent. The historical market environment in the United States contributed greatly to the idea that a 4 percent withdrawal rate in retirement is sufficiently conservative to prevent asset depletion. Forward-looking estimates using a similar methodology suggest that the 4 percent rule should not continue to be treated as safe.
Following the methodology outlined in Pfau (2012) who used historical averages, a 30-year retirement, and 10 percent acceptable failure rate, the maximum sustainable withdrawal rate was 4.3 percent with an asset allocation of 45 percent stocks and 55 percent bonds. When using current market conditions of a −1.4 percent real bond return and the historical equity premium, the maximum sustainable withdrawal rate would drop to 2.5 percent with an almost identical optimal asset allocation. This withdrawal rate is 1.8 percent less than found with historical averages.
Conclusion
Estimating a withdrawal rate from retirement assets that will preserve capital over a long life requires assumptions about future asset yields. Current bond yields are at historical lows, and the R-squared between current and five-year and 10-year bond yields is about 0.90. Research shows returns during the first 10 years of retirement have an inordinately large impact on failure rates. In this study, we estimated the impact of current low bond yields on two retirement planning scenarios: one where estimates of future bond returns are equal to or near the most recent yields and the other where bond yields and returns revert back to their historical mean.
Results suggest that failure rates are surprisingly sensitive to a downward adjustment of bond returns. With no real bond yield, hypothetical retirees experience a one in three chance of running out of money after 30 years with a 50 percent stock portfolio. If the current negative real yields on five-year bond investments persist, the probability that one will run out of money is greater than 50 percent. These estimates also assume that the historical equity premium will not change and that there are no asset management fees.
Reversion to historical mean bond returns will not save the 4 percent rule. Hypothetical retirees experience a nearly 20 percent probability of running out of money even if bond returns revert back to the historical mean after only five years with a 50 percent stock portfolio. If the mean returns on bonds revert in 10 years, the failure rate still rises to 33 percent. These estimates do not take into account the capital loss to a bond portfolio if interest rates rise. If a planner increases the duration of bonds early in retirement to reach for a greater bond yield, this reversion can have a particularly negative impact on failure rates.
As Pfau (2010) showed, the demonstrated success of the 4 percent rule is partly an anomaly of U.S. market returns during the 20th century. In most other countries, sustainable initial withdrawal rates fell below 4 percent. This study indicates that there is nothing inherently safe about the 4 percent rule. When withdrawing from a portfolio of volatile assets, surprises may happen. This study demonstrates that when financial planners recalibrate assumptions for Monte Carlo simulations to market conditions facing retirees in 2013, the 4 percent rule is anything but safe.
This research also shows that a 2.5 percent real withdrawal rate will result in an estimated 30-year failure rate of 10 percent. Few clients will be satisfied spending such a small amount in retirement.
To end on a positive note, we believe it is possible to boost optimal withdrawal rates by incorporating assets that provide a mortality credit and longevity protection. Pfau (2013), for example, estimated that combining stocks with single premium immediate annuities, rather than bonds, provides an opportunity for clients to achieve the dual goals of meeting desired lifestyle spending and preserving a larger reserve of financial assets. In the absence of some added income protection, there is a high likelihood that low yields will require planners to rethink the safety of a traditional investment-based retirement income plan.
In following the traditional retirement income shortfall methodology, we have illustrated the shortfall danger of following a strict real consumption path over 30 years in a low-yield environment. To be consistent with the withdrawal rate methodology for previous studies, we have used the five-year implied real return from TIPS. As authors, we are not necessarily suggesting this is the most likely forecast of expected returns over the next 30 years; however, we feel it is important to place our results in the context of other papers that use this approach.
This methodology also ignores the possibility that a retiree can respond to poor market returns by dynamically adjusting consumption over time (Guyton 2004), and the opportunity cost of unspent wealth among those who do not have a strong bequest motive (Finke, Pfau, and Williams 2012). Scott, Sharpe, and Watson (2009) also pointed out that a 4 percent rule of thumb ignores how much a retiree would ideally adjust spending during retirement to maximize enjoyment. An ideal retirement income plan should emphasize not only shortfall risk from an investment portfolio, but also should consider retiree preferences and a broader range of retirement income products.
Endnotes
- A recent working paper by Pflueger and Viceira (2012) of the Harvard Business School argues that TIPS rates also include an illiquidity premium of roughly 70 basis points above comparable short-term bond investments. This is because TIPS are traded less frequently than other government bonds. A less liquid market implies that current TIPS rates underestimate the market’s willingness to trade real consumption over time (the rate is higher than it would be in the absence of an illiquidity premium).?
- Using longer duration TIPS yields would incorporate an illiquidity and risk premium that will bias yields upward relative to bond portfolios used in previous Monte Carlo analyses.
- The assumption that the equity risk premium will equal the historical premium may be overly optimistic. Current equity valuations scaled by 10-year corporate earnings are about 30 percent higher than their historical average. A recent review of the equity premium by New York University professor Aswath Damodaran (2012) finds strong empirical evidence that current valuation is a much better predictor of future equity returns than the historical premium. A 2010 survey of chief financial officers found an average equity premium estimate of 3 percent (Graham and Harvey, 2010).
References
Arnott, Robert D., and Denis B. Chaves. 2012. “Demographic Changes, Financial Markets, and the Economy.” Financial Analysts Journal 68 (1): 23–46.
Bakshi, Gurdip S., and Zhiwu Chen. 1994. “Baby Boom, Population Aging, and Capital Markets.” Journal of Business 67 (2): 165–202.
Bengen, William P. 1994. “Determining Withdrawal Rates Using Historical Data.” Journal of Financial Planning 7 (4): 171–180.
Bernanke, Ben. 2013. “Long-Term Interest Rates.” Board of Governors of the Federal Reserve Board Speech, March 1, www.federalreserve.gov/newsevents/speech/bernanke20130301a.htm.
Blanchett, David M., and Brian C. Blanchett. 2008. “Data Dependence and Sustainable Real Withdrawal Rates.” Journal of Financial Planning 21 (9): 70–85.
Cooley, Philip L., Hubbard, Carl M. and Daniel T. Walz. 1998. “Retirement Savings: Choosing a Withdrawal Rate that is Sustainable.” Journal of the American Association of Individual Investors 20 (2): 16–21.
Damodaran, Aswath. 2012. “Equity Risk Premiums: Determinants, Estimation and Implications–The 2012 Edition.” Working Paper, http://people.stern.nyu.edu/adamodar/pdfiles/papers/ERP2012.pdf.
Feldstein, Martin. 2013. “The Fed’s Dangerous Direction.” The Wall Street Journal, January 3 print edition.
Finke, Michael, Wade Pfau, and Duncan Williams. 2012. “Spending Flexibility and Safe Withdrawal Rates.” Journal of Financial Planning 25 (3): 44–51.
Graham, John R., and Campbell R. Harvey. 2010. “The Equity Risk Premium in 2010” SSRN Working Paper, http://papers.ssrn.com/sol3/papers.cfm?abstract_id=1654026.
Guyton, Jonathan T. 2004. “Decision Rules and Portfolio Management for Retirees: Is the ‘Safe’ Initial Withdrawal Rate Too Safe?” Journal of Financial Planning 17 (10): 50–58.
Kitces, Michael E. 2008. “Resolving the Paradox—Is the Safe Withdrawal Rate Sometimes Too Safe?” The Kitces Report. www.kitces.com/assets/pdfs/Kitces_Report_May_2008.pdf.
Milevsky, Moshe, and Anna Abaimova. 2006. “Risk Management During Retirement,” in Retirement Income Redesigned: Master Plans for Distribution, edited by Harold Evensky and Deena Katz, 163–184. New York: Bloomberg Press.
Pfau, Wade D. 2010. “An International Perspective on Safe Withdrawal Rates: The Demise of the 4 Percent Rule?” Journal of Financial Planning 23 (12): 52–61.
Pfau, Wade D. 2011a. “Can We Predict the Sustainable Withdrawal Rate for New Retirees?” Journal of Financial Planning 24 (8): 40–47.
Pfau, Wade D. 2011b. “Will 2000-Era Retirees Experience the Worst Retirement Outcomes in U.S. History? A Progress Report after 10 Years.” Journal of Investing 20 (4): 117–131.
Pfau, Wade D. 2011c. “Retirement Withdrawal Rates and Portfolio Success Rates: What Can the Historical Record Teach Us?” Retirement Management Journal 1 (2): 49–55.
Pfau, W. D. 2012. “Capital Market Expectations, Asset Allocation, and Safe Withdrawal Rates.” Journal of Financial Planning 25 (1): 36–43.
Pfau, W. D. 2013. “A Broader Framework for Determining an Efficient Frontier for Retirement Income.” Journal of Financial Planning 26 (2): 44–51.
Pflueger, Carolin E., and Luis M. Viceira. 2012. “An Empirical Decomposition of Risk and Liquidity in Nominal and Inflation-Indexed Government Bonds.” Harvard Business School Working Paper, www.people.hbs.edu/lviceira/PV-TIPS-20120410-ALL.pdf.
Scott, Jason S., William F. Sharpe, and John G. Watson. 2009. “The 4% Rule at What Price?” Journal of Investment Management 7 (3): 31–48.
Spitzer, John J., Jeffrey C. Strieter, and Sandeep Singh. 2007. “Guidelines for Withdrawal Rates and Portfolio Safety During Retirement.” Journal of Financial Planning 20 (10): 52–59.
van den End, Jan Willem. 2011. “Statistical Evidence on the Mean Reversion of Interest Rates. DNB Working Paper no. 284. Netherlands Central Bank Research Department.
Vasicek, Oldrich. 1977. “An Equilibrium Characterization of the Term Structure.” Journal of Financial Economics 5 (2): 177–188.
Citation
Finke, Michael, Wade D. Pfau, and David M. Blanchett. 2013. “The 4 Percent Rule Is Not Safe in a Low-Yield World.” Journal of Financial Planning 26 (6): 46–55.